Sections of the site
Editor's Choice:
- Pericardial syntopy. Topography of the pericardium. Diverticula and pericardial cysts
- Media and mass culture Characteristics of mass culture and examples
- The nature of modern wars and armed conflicts in brief Conventional and illegitimate wars
- Why do you dream of a white wedding dress: decoding the image from the dream book
- Arkady Moreinis, Glavstart: “I work with startups, not sell boxes of coffee General Director of the Glavstart company”
- A box of quality problems in physics and electricity How does a naval galvanic impact mine work?
- Sergey Cheremin: “A compatriot is someone who shares the cultural values of the Russian world”
- “A pilot lives only in flight”
- Spring is the time to bloom quotes
- How to get rid of uterine polyps?
Advertising
| How to reduce algebraic fractions with powers. Multiplying, dividing and reducing algebraic fractions |
|
This article continues the topic of converting algebraic fractions: consider such an action as reducing algebraic fractions. Let's define the term itself, formulate a reduction rule and analyze practical examples. Yandex.RTB R-A-339285-1 The meaning of reducing an algebraic fractionIn materials about common fractions, we looked at its reduction. We defined reducing a fraction as dividing its numerator and denominator by a common factor. Reducing an algebraic fraction is a similar operation. Definition 1 Reducing an algebraic fraction is the division of its numerator and denominator by a common factor. In this case, in contrast to the reduction of an ordinary fraction (the common denominator can only be a number), the common factor of the numerator and denominator of an algebraic fraction can be a polynomial, in particular, a monomial or a number. For example, the algebraic fraction 3 x 2 + 6 x y 6 x 3 y + 12 x 2 y 2 can be reduced by the number 3, resulting in: x 2 + 2 x y 6 x 3 · y + 12 · x 2 · y 2 . We can reduce the same fraction by the variable x, and this will give us the expression 3 x + 6 y 6 x 2 y + 12 x y 2. It is also possible to reduce a given fraction by a monomial 3 x or any of the polynomials x + 2 y, 3 x + 6 y , x 2 + 2 x y or 3 x 2 + 6 x y. The ultimate goal of reducing an algebraic fraction is a fraction of a simpler form, in best case scenario– irreducible fraction. Are all algebraic fractions subject to reduction?Again, from materials on ordinary fractions, we know that there are reducible and irreducible fractions. Irreducible fractions are fractions that do not have common factors in the numerator and denominator other than 1. It’s the same with algebraic fractions: they may have common factors in the numerator and denominator, or they may not. The presence of common factors allows you to simplify the original fraction through reduction. When there are no common factors, it is impossible to optimize a given fraction using the reduction method. In general cases, given the type of fraction it is quite difficult to understand whether it can be reduced. Of course, in some cases the presence of a common factor between the numerator and denominator is obvious. For example, in the algebraic fraction 3 x 2 3 y it is quite clear that the common factor is the number 3. In the fraction - x · y 5 · x · y · z 3 we also immediately understand that it can be reduced by x, or y, or x · y. And yet, much more often there are examples of algebraic fractions, when the common factor of the numerator and denominator is not so easy to see, and even more often, it is simply absent. For example, we can reduce the fraction x 3 - 1 x 2 - 1 by x - 1, while the specified common factor is not present in the entry. But the fraction x 3 - x 2 + x - 1 x 3 + x 2 + 4 · x + 4 cannot be reduced, since the numerator and denominator do not have a common factor. Thus, the question of determining the reducibility of an algebraic fraction is not so simple, and it is often easier to work with a fraction of a given form than to try to find out whether it is reducible. In this case, such transformations take place that in particular cases make it possible to determine the common factor of the numerator and denominator or to draw a conclusion about the irreducibility of a fraction. We will examine this issue in detail in the next paragraph of the article. Rule for reducing algebraic fractionsRule for reducing algebraic fractions consists of two sequential actions:
The most convenient method for finding common denominators is to factor the polynomials present in the numerator and denominator of a given algebraic fraction. This allows you to immediately clearly see the presence or absence of common factors. The very action of reducing an algebraic fraction is based on the main property of an algebraic fraction, expressed by the equality undefined, where a, b, c are some polynomials, and b and c are non-zero. The first step is to reduce the fraction to the form a · c b · c, in which we immediately notice the common factor c. The second step is to perform a reduction, i.e. transition to a fraction of the form a b . Typical examplesDespite some obviousness, let us clarify about special case when the numerator and denominator of an algebraic fraction are equal. Similar fractions are identically equal to 1 on the entire ODZ of the variables of this fraction: 5 5 = 1 ; - 2 3 - 2 3 = 1 ; x x = 1 ; - 3, 2 x 3 - 3, 2 x 3 = 1; 1 2 · x - x 2 · y 1 2 · x - x 2 · y ; Because the common fractions are a special case of algebraic fractions, let us recall how their reduction is carried out. The natural numbers written in the numerator and denominator are factored into prime factors, then the common factors are canceled (if any). For example, 24 1260 = 2 2 2 3 2 2 3 3 5 7 = 2 3 5 7 = 2 105 The product of simple identical factors can be written as powers, and in the process of reducing a fraction, use the property of dividing powers with identical bases. Then the above solution would be: 24 1260 = 2 3 3 2 2 3 2 5 7 = 2 3 - 2 3 2 - 1 5 7 = 2 105 (numerator and denominator divided by a common factor 2 2 3). Or for clarity, based on the properties of multiplication and division, we give the solution the following form: 24 1260 = 2 3 3 2 2 3 2 5 7 = 2 3 2 2 3 3 2 1 5 7 = 2 1 1 3 1 35 = 2 105 By analogy, the reduction of algebraic fractions is carried out, in which the numerator and denominator have monomials with integer coefficients. Example 1 The algebraic fraction is given - 27 · a 5 · b 2 · c · z 6 · a 2 · b 2 · c 7 · z. It needs to be reduced. Solution It is possible to write the numerator and denominator of a given fraction as a product of simple factors and variables, and then carry out the reduction: 27 · a 5 · b 2 · c · z 6 · a 2 · b 2 · c 7 · z = - 3 · 3 · 3 · a · a · a · a · a · b · b · c · z 2 · 3 · a · a · b · b · c · c · c · c · c · c · c · z = = - 3 · 3 · a · a · a 2 · c · c · c · c · c · c = - 9 a 3 2 c 6 However, a more rational way would be to write the solution as an expression with powers: 27 · a 5 · b 2 · c · z 6 · a 2 · b 2 · c 7 · z = - 3 3 · a 5 · b 2 · c · z 2 · 3 · a 2 · b 2 · c 7 · z = - 3 3 2 · 3 · a 5 a 2 · b 2 b 2 · c c 7 · z z = = - 3 3 - 1 2 · a 5 - 2 1 · 1 · 1 c 7 - 1 · 1 = · - 3 2 · a 3 2 · c 6 = · - 9 · a 3 2 · c 6 . Answer:- 27 a 5 b 2 c z 6 a 2 b 2 c 7 z = - 9 a 3 2 c 6 When the numerator and denominator of an algebraic fraction contain fractional numerical coefficients, there are two possible ways of further action: either divide these fractional coefficients separately, or first get rid of the fractional coefficients by multiplying the numerator and denominator by a certain natural number. The last transformation is carried out due to the basic property of an algebraic fraction (you can read about it in the article “Reducing an algebraic fraction to a new denominator”). Example 2 The given fraction is 2 5 x 0, 3 x 3. It needs to be reduced. Solution It is possible to reduce the fraction this way: 2 5 x 0, 3 x 3 = 2 5 3 10 x x 3 = 4 3 1 x 2 = 4 3 x 2 Let's try to solve the problem differently, having first gotten rid of fractional coefficients - multiply the numerator and denominator by the least common multiple of the denominators of these coefficients, i.e. on LCM (5, 10) = 10. Then we get: 2 5 x 0, 3 x 3 = 10 2 5 x 10 0, 3 x 3 = 4 x 3 x 3 = 4 3 x 2. Answer: 2 5 x 0, 3 x 3 = 4 3 x 2 When we reduce algebraic fractions general view, in which the numerators and denominators can be either monomials or polynomials, there may be a problem when the common factor is not always immediately visible. Or moreover, it simply does not exist. Then, to determine the common factor or record the fact of its absence, the numerator and denominator of the algebraic fraction are factored. Example 3 The rational fraction 2 · a 2 · b 2 + 28 · a · b 2 + 98 · b 2 a 2 · b 3 - 49 · b 3 is given. It needs to be reduced. Solution Let's factor the polynomials in the numerator and denominator. Let's put it out of brackets: 2 a 2 b 2 + 28 a b 2 + 98 b 2 a 2 b 3 - 49 b 3 = 2 b 2 (a 2 + 14 a + 49) b 3 (a 2 - 49) We see that the expression in parentheses can be converted using abbreviated multiplication formulas: 2 b 2 (a 2 + 14 a + 49) b 3 (a 2 - 49) = 2 b 2 (a + 7) 2 b 3 (a - 7) (a + 7) It is clearly seen that it is possible to reduce a fraction by a common factor b 2 (a + 7). Let's make a reduction: 2 b 2 (a + 7) 2 b 3 (a - 7) (a + 7) = 2 (a + 7) b (a - 7) = 2 a + 14 a b - 7 b Let us write a short solution without explanation as a chain of equalities: 2 a 2 b 2 + 28 a b 2 + 98 b 2 a 2 b 3 - 49 b 3 = 2 b 2 (a 2 + 14 a + 49) b 3 (a 2 - 49) = = 2 b 2 (a + 7) 2 b 3 (a - 7) (a + 7) = 2 (a + 7) b (a - 7) = 2 a + 14 a b - 7 b Answer: 2 a 2 b 2 + 28 a b 2 + 98 b 2 a 2 b 3 - 49 b 3 = 2 a + 14 a b - 7 b. It happens that common factors are hidden by numerical coefficients. Then, when reducing fractions, it is optimal to put the numerical factors at higher powers of the numerator and denominator out of brackets. Example 4 Given the algebraic fraction 1 5 · x - 2 7 · x 3 · y 5 · x 2 · y - 3 1 2 . It is necessary to reduce it if possible. Solution At first glance, the numerator and denominator do not have a common denominator. However, let's try to convert the given fraction. Let's take out the factor x in the numerator: 1 5 x - 2 7 x 3 y 5 x 2 y - 3 1 2 = x 1 5 - 2 7 x 2 y 5 x 2 y - 3 1 2 Now you can see some similarity between the expression in brackets and the expression in the denominator due to x 2 y . Let us take out the numerical coefficients of the higher powers of these polynomials: x 1 5 - 2 7 x 2 y 5 x 2 y - 3 1 2 = x - 2 7 - 7 2 1 5 + x 2 y 5 x 2 y - 1 5 3 1 2 = = - 2 7 x - 7 10 + x 2 y 5 x 2 y - 7 10 Now the common factor becomes visible, we carry out the reduction: 2 7 x - 7 10 + x 2 y 5 x 2 y - 7 10 = - 2 7 x 5 = - 2 35 x Answer: 1 5 x - 2 7 x 3 y 5 x 2 y - 3 1 2 = - 2 35 x . Let us emphasize that the skill of reducing rational fractions depends on the ability to factor polynomials. If you notice an error in the text, please highlight it and press Ctrl+Enter Using fractions, the same part of a whole object can be written in different ways. Half of the circle is shaded in the picture So all these fractions are equal. For convenience, the additional factor is written on the slash on the right above the fraction.
Let's go back to our fractions again and write them in a different order.
A fraction equal to a given one can be obtained if the numerator and denominator of the fraction are simultaneously divided by the same number that is not equal to zero. This conversion of a fraction is called reducing a fraction. Reducing a fraction is usually written as follows. The numerator and denominator are crossed out, and the results of division (quotients) of the numerator and denominator by the same number are written next to them. Keep the number by which the numerator and denominator are divided in mind.
In our example, we reduced (that is, divided both the numerator and denominator) a fraction by two, which we kept in mind. Fraction reduction can be done sequentially.
The main property of a fractionLet us formulate the main property of a fraction. If the numerator and denominator of a fraction are multiplied or divided by the same number that is not equal to zero, you get a fraction equal to the given one. Let us write this property in the form of literal expressions.
Reducing fractions, rules and examples of reducing fractions.In this article we will look in detail at how reducing fractions. First, let's discuss what is called reducing a fraction. After this, let's talk about reducing a reducible fraction to an irreducible form. Next we will obtain the rule for reducing fractions and, finally, consider examples of the application of this rule. Page navigation. What does it mean to reduce a fraction?We know that ordinary fractions are divided into reducible and irreducible fractions. You can guess from the names that reducible fractions can be reduced, but irreducible fractions cannot. What does it mean to reduce a fraction? Reduce fraction- this means dividing its numerator and denominator by their positive and non-unity common divisor. It is clear that as a result of reducing a fraction, a new fraction with a smaller numerator and denominator is obtained, and, due to the basic property of the fraction, the resulting fraction is equal to the original one. For example, let's reduce the common fraction 8/24 by dividing its numerator and denominator by 2. In other words, let's reduce the fraction 8/24 by 2. Since 8:2=4 and 24:2=12, this reduction results in the fraction 4/12, which is equal to the original fraction 8/24 (see equal and unequal fractions). As a result, we have . Reducing ordinary fractions to irreducible formUsually ultimate goal reducing a fraction is to obtain an irreducible fraction that is equal to the original reducible fraction. This goal can be achieved by reducing the original reducible fraction by the greatest common divisor of its numerator and denominator. As a result of such a reduction, an irreducible fraction is always obtained. Indeed, a fraction So, reducing a common fraction to an irreducible form consists of dividing the numerator and denominator of the original reducible fraction by their gcd. Let's look at an example, for which we return to the fraction 8/24 and reduce it by the greatest common divisor of the numbers 8 and 24, which is equal to 8. Since 8:8=1 and 24:8=3, we come to the irreducible fraction 1/3. So, . Note that the phrase “reduce a fraction” often means reducing the original fraction to its irreducible form. In other words, reducing a fraction very often refers to dividing the numerator and denominator by their greatest common factor (rather than by any common factor). How to reduce a fraction? Rules and examples of reducing fractionsAll that remains is to look at the rule for reducing fractions, which explains how to reduce a given fraction. Rule for reducing fractions consists of two steps:
Let's sort it out example of reducing a fraction according to the stated rule. www.cleverstudents.ru Reducing fractions. What does it mean to reduce a fraction?Reducing fractions is necessary in order to reduce the fraction to more simple view, for example, in the answer obtained as a result of solving an expression. Reducing fractions, definition and formula.What is reducing fractions? What does it mean to reduce a fraction? Definition: Formula for reducing fractions basic properties of rational numbers. Let's look at an example: Solution: Answer: after reduction we got the fraction \(\frac\). According to the basic property of rational numbers, the original and resulting fractions are equal. How to reduce fractions? Reducing a fraction to its irreducible form.To get an irreducible fraction as a result, we need find the greatest common divisor (GCD) for the numerator and denominator of the fraction. There are several ways to find GCD; in the example we will use the decomposition of numbers into prime factors. Get the irreducible fraction \(\frac\). Solution: The rule for reducing a fraction to an irreducible form.
Example: Solution: Answer: \(\frac \) is an irreducible fraction. Reducing improper fractions.How to reduce an improper fraction? Let's look at an example: Solution: Reducing mixed fractions.Mixed fractions follow the same rules as ordinary fractions. The only difference is that we can do not touch the whole part, but reduce the fractional part or mixed fraction convert to an improper fraction, reduce and convert back to a proper fraction. Let's look at an example: Solution: Second way: Related questions:
Solution: What numbers can you reduce a fraction by?
Let's write the numbers 100 and 150 into prime factors. We got the irreducible fraction \(\frac \). But it is not necessary to always divide by gcd; an irreducible fraction is not always needed; you can reduce the fraction by a simple divisor of the numerator and denominator. For example, the number 100 and 150 have a common divisor of 2. Let's reduce the fraction \(\frac \) by 2. We got the reducible fraction \(\frac\). What fractions can be reduced?
Example: These two fractions are equal. Let's take a closer look at the fraction \(\frac \): Two fractions are equal if and only if one of them is obtained by reducing the other fraction by the common factor of the numerator and denominator. Example: Operations with ordinary fractionsFraction expansion. Reducing a fraction. Comparing fractions. Reduction to a common denominator. Addition and subtraction fractions. Multiplying fractions. Division of fractions . Fraction expansion. The value of a fraction does not change if you multiply its numerator and denominator by the same number other than zero. expansion of a fraction. For example,
Reducing a fraction. The value of a fraction does not change if you divide its numerator and denominator by the same number other than zero.. This transformation is called reducing a fraction. For example,
Comparing fractions. Of two fractions with the same numerators, the one whose denominator is smaller is greater:
Of two fractions with the same denominator, the one whose numerator is greater is greater:
To compare fractions that have different numerators and denominators, you need to expand them to bring them to a common denominator. EXAMPLE Compare two fractions: Let's expand the first fraction by the denominator of the second, and the second by the denominator of the first: The transformation used here is called reducing fractions to a common denominator. Adding and subtracting fractions. If the denominators of the fractions are the same, then in order to add the fractions, you need to add their numerators, and in order to subtract the fractions, you need to subtract their numerators (in the same order). The resulting sum or difference will be the numerator of the result; the denominator will remain the same. If the denominators of the fractions are different, you must first reduce the fractions to a common denominator. When adding mixed numbers, their whole and fractional parts are added separately. When subtracting mixed numbers, we recommend first converting them to improper fractions, then subtracting one from the other, and then converting the result again, if necessary, to mixed number form.
Multiplying fractions. To multiply a number by a fraction means to multiply it by the numerator and divide the product by the denominator. Therefore we have general rule multiplying fractions: to multiply fractions, you need to multiply their numerators and denominators separately and divide the first product by the second. EXAMPLE Dividing fractions. In order to divide a number by a fraction, you need to multiply this number by the reciprocal fraction. This rule follows from the definition of division (see the section “Arithmetic Operations”). EXAMPLE Multiplying and dividing fractionsLast time we learned how to add and subtract fractions (see lesson “Adding and Subtracting Fractions”). The most difficult part of those actions was bringing fractions to a common denominator. Now it's time to deal with multiplication and division. The good news is that these operations are even simpler than addition and subtraction. First, let's consider the simplest case, when there are two positive fractions without a separated integer part. To multiply two fractions, you must multiply their numerators and denominators separately. The first number will be the numerator of the new fraction, and the second will be the denominator. To divide two fractions, you need to multiply the first fraction by the “inverted” second fraction. From the definition it follows that dividing fractions reduces to multiplication. To “flip” a fraction, just swap the numerator and denominator. Therefore, throughout the lesson we will mainly consider multiplication. As a result of multiplication, a reducible fraction can arise (and often does arise) - it, of course, must be reduced. If after all the reductions the fraction turns out to be incorrect, the whole part should be highlighted. But what definitely won't happen with multiplication is reduction to a common denominator: no criss-cross methods, greatest factors and least common multiples.
Multiplying fractions with whole parts and negative fractionsIf present in fractions whole part, they must be converted into incorrect ones - and only then multiplied according to the schemes outlined above. If there is a minus in the numerator of a fraction, in the denominator or in front of it, it can be taken out of the multiplication or removed altogether according to the following rules: Until now, these rules have only been encountered when adding and subtracting negative fractions, when it was necessary to get rid of the whole part. For a work, they can be generalized in order to “burn” several disadvantages at once: Task. Find the meaning of the expression: We convert all fractions to improper ones, and then take the minuses out of the multiplication. We multiply what is left according to the usual rules. We get:
Let me remind you once again that the minus that appears in front of a fraction with a highlighted whole part refers specifically to the entire fraction, and not just to its whole part (this applies to the last two examples). Also pay attention to negative numbers: when multiplying, they are enclosed in parentheses. This is done in order to separate the minuses from the multiplication signs and make the entire notation more accurate. Reducing fractions on the flyMultiplication is a very labor-intensive operation. The numbers here turn out to be quite large, and to simplify the problem, you can try to reduce the fraction further before multiplication. Indeed, in essence, the numerators and denominators of fractions are ordinary factors, and, therefore, they can be reduced using the basic property of a fraction. Take a look at the examples:
By definition we have:
In all examples, the numbers that have been reduced and what remains of them are marked in red. Please note: in the first case, the multipliers were reduced completely. In their place there remain units that, generally speaking, need not be written. In the second example, it was not possible to achieve a complete reduction, but the total amount of calculations still decreased. However, never use this technique when adding and subtracting fractions! Yes, sometimes there are similar numbers that you just want to reduce. Here, look: You can't do that! The error occurs because when adding, the numerator of a fraction produces a sum, not a product of numbers. Therefore, it is impossible to apply the main property of a fraction, since in this property we're talking about specifically about multiplying numbers. There are simply no other reasons for reducing fractions, so the correct solution to the previous problem looks like this: As you can see, the correct answer turned out to be not so beautiful. In general, be careful. In this lesson we will study the basic property of a fraction, find out which fractions are equal to each other. We'll learn to reduce fractions, determine whether a fraction is reducible or not, practice reducing fractions, and learn when to use a contraction and when not to. Lorem ipsum dolor sit amet, consectetur adipisicing elit. Adipisci autem beatae consectetur corporis dolores ea, eius, esse id illo inventore iste mollitia nemo nesciunt nisi obcaecati optio similique tempore voluptate! Adipisci alias assumenda consequatur cupiditate, ex id minima quam rem sint vitae? Animi dolores earum enim fugit magni nihil odit provident quaerat. Aliquid aspernatur eos esse magnam maiores necessitatibus, nulla? This information is available to registered users The main property of a fractionImagine this situation. At the table 3 person and 5 apples Share 5 apples for three. Everyone gets \(\mathbf(\frac(5)(3))\) apples. And at the next table 3 person and too 5 apples Each again \(\mathbf(\frac(5)(3))\) In total 10 apples 6 Human. Each one \(\mathbf(\frac(10)(6))\) But it's the same thing. \(\mathbf(\frac(5)(3) = \frac(10)(6))\) These fractions are equivalent. You can double the number of people and double the number of apples. The result will be the same. In mathematics it is formulated like this: If the numerator and denominator of a fraction are multiplied or divided by the same number (not equal to 0), then the new fraction will be equal to the original. This property is sometimes called " main property of a fraction ». $$\mathbf(\frac(a)(b) = \frac(a\cdot c)(b\cdot c) = \frac(a:d)(b:d))$$ For example, The path from city to village - 14 km. We walk along the road and determine the distance traveled by kilometer markers. Having walked six columns, six kilometers, we understand that we have covered \(\mathbf(\frac(6)(14))\) distance. But if we don’t see the poles (maybe they weren’t installed), we can calculate the path using the electric poles along the road. Their 40 pieces for every kilometer. That is, in total 560 all the way. Six kilometers - \(\mathbf(6\cdot40 = 240)\) pillars. That is, we have passed 240 from 560 pillars-\(\mathbf(\frac(240)(560))\) \(\mathbf(\frac(6)(14) = \frac(240)(560))\) Example 1 Mark a point with coordinates ( 5; 7 ) on the coordinate plane XOY. It will correspond to the fraction \(\mathbf(\frac(5)(7))\) Connect the origin of coordinates to the resulting point. Construct another point that has coordinates twice the previous ones. What fraction did you get? Will they be equal? Solution A fraction on the coordinate plane can be marked with a dot. To represent the fraction \(\mathbf(\frac(5)(7))\), mark the point with the coordinate 5 along the axis Y And 7 along the axis X. Let's draw a straight line from the origin through our point. The point corresponding to the fraction \(\mathbf(\frac(10)(14))\) will also lie on the same line They are equivalent: \(\mathbf(\frac(5)(7) = \frac(10)(14))\) In this article we will look at basic operations with algebraic fractions:
Let's start with reduction of algebraic fractions. It would seem that, algorithm obvious. To reduce algebraic fractions, need to 1. Factor the numerator and denominator of the fraction. 2. Reduce equal factors. However, schoolchildren often make the mistake of “reducing” not the factors, but the terms. For example, there are amateurs who “reduce” fractions by and get as a result , which, of course, is not true. Let's look at examples: 1.
Reduce fraction: 1. Let us factorize the numerator using the formula of the square of the sum, and the denominator using the formula of the difference of squares
2. Divide the numerator and denominator by
2.
Reduce fraction: 1. Let's factorize the numerator. Since the numerator contains four terms, we use grouping. 2. Let's factorize the denominator. We can also use grouping. 3. Let's write down the fraction that we got and reduce the same factors:
Multiplying algebraic fractions. When multiplying algebraic fractions, we multiply the numerator by the numerator, and multiply the denominator by the denominator.
Let's look at examples: 3. Simplify the expression:
1. Let’s write the product of fractions: in the numerator the product of the numerators, and in the denominator the product of the denominators:
2. Let's factorize each bracket:
Now we need to reduce the same factors. Note that the expressions and differ only in sign:
So, We divide algebraic fractions according to the following rule:
We see that dividing fractions comes down to multiplying, and multiplication ultimately comes down to reducing fractions. Let's look at an example: 4. Simplify the expression:
So, we already know that the numerator and denominator of a fraction can be multiplied and divided by the same number, the fraction will not change. Let's consider three approaches: Approach one. To reduce, divide the numerator and denominator by a common divisor. Let's look at examples:
Let's shorten: In the examples given, we immediately see which divisors to take for reduction. The process is simple - we go through 2,3,4,5 and so on. In most school course examples, this is quite enough. But if it’s a fraction:
Here the process of selecting divisors can take a long time;). Of course, such examples are outside the school curriculum, but you need to be able to cope with them. Below we will look at how this is done. For now, let's get back to the downsizing process. As discussed above, in order to reduce a fraction, we divided by the common divisor(s) we determined. Everything is correct! One has only to add signs of divisibility of numbers: - if the number is even, then it is divisible by 2. - if a number from the last two digits is divisible by 4, then the number itself is divisible by 4. — if the sum of the digits that make up the number is divisible by 3, then the number itself is divisible by 3. For example, 125031, 1+2+5+0+3+1=12. Twelve is divisible by 3, so 123031 is divisible by 3. - if the number ends with 5 or 0, then the number is divisible by 5. — if the sum of the digits that make up the number is divisible by 9, then the number itself is divisible by 9. For example, 625032 =.> 6+2+5+0+3+2=18. Eighteen is divisible by 9, which means 623032 is divisible by 9. Second approach. To put it briefly, in fact, the whole action comes down to factoring the numerator and denominator and then reducing equal factors in the numerator and denominator (this approach is a consequence of the first approach):
Visually, in order to avoid confusion and mistakes, equal factors are simply crossed out. Question - how to factor a number? It is necessary to determine all divisors by searching. This is a separate topic, it is not complicated, look up the information in a textbook or on the Internet. You will not encounter any great problems with factoring numbers that are present in school fractions. Formally, the reduction principle can be written as follows:
Approach three. Here is the most interesting thing for the advanced and those who want to become one. Let's reduce the fraction 143/273. Try it yourself! Well, how did it happen quickly? Now look! We turn it over (we change places of the numerator and denominator). We divide the resulting fraction with a corner and convert it into a mixed number, that is, we select the whole part:
It's already easier. We see that the numerator and denominator can be reduced by 13:
Now don’t forget to flip the fraction back again, let’s write down the whole chain:
Checked - it takes less time than searching through and checking divisors. Let's return to our two examples:
First. Divide with a corner (not on a calculator), we get:
This fraction is simpler, of course, but the reduction is again a problem. Now we separately analyze the fraction 1273/1463 and turn it over:
It's easier here. We can consider a divisor such as 19. The rest are not suitable, this is clear: 190:19 = 10, 1273:19 = 67. Hurray! Let's write down:
Next example. Let's shorten it to 88179/2717. Divide, we get:
Separately, we analyze the fraction 1235/2717 and turn it over:
We can consider a divisor such as 13 (up to 13 is not suitable): Numerator 247:13=19 Denominator 1235:13=95 *During the process we saw another divisor equal to 19. It turns out that:
Now we write down the original number: And it doesn’t matter what is larger in the fraction - the numerator or the denominator, if it is the denominator, then we turn it over and act as described. This way we can reduce any fraction; the third approach can be called universal. Of course, the two examples discussed above are not simple examples. Let's try this technology on the “simple” fractions we have already considered:
Two quarters. Seventy-two sixties. The numerator is greater than the denominator; there is no need to reverse it: Of course, the third approach was applied to such simple examples just as an alternative. The method, as already said, is universal, but not convenient and correct for all fractions, especially for simple ones. The variety of fractions is great. It is important that you understand the principles. There is simply no strict rule for working with fractions. We looked, figured out how it would be more convenient to act, and moved forward. With practice, skill will come and you will crack them like seeds. Conclusion: If you see a common divisor(s) for the numerator and denominator, use them to reduce. If you know how to quickly factor a number, then factor the numerator and denominator, then reduce. If you can’t determine the common divisor, then use the third approach. *To reduce fractions, it is important to master the principles of reduction, understand the basic property of a fraction, know approaches to solving, and be extremely careful when making calculations. And remember! It is customary to reduce a fraction until it stops, that is, reduce it as long as there is a common divisor. Sincerely, Alexander Krutitskikh. |
Popular:
New
- Media and mass culture Characteristics of mass culture and examples
- The nature of modern wars and armed conflicts in brief Conventional and illegitimate wars
- Why do you dream of a white wedding dress: decoding the image from the dream book
- Arkady Moreinis, Glavstart: “I work with startups, not sell boxes of coffee General Director of the Glavstart company”
- A box of quality problems in physics and electricity How does a naval galvanic impact mine work?
- Sergey Cheremin: “A compatriot is someone who shares the cultural values of the Russian world”
- “A pilot lives only in flight”
- Spring is the time to bloom quotes
- How to get rid of uterine polyps?
- Riddles, proverbs, sayings, stories and poems about linden Properties of linden tree





 , where "a", "b" and "k" are natural numbers.
, where "a", "b" and "k" are natural numbers. is irreducible, since from the properties of GCD it is known that
is irreducible, since from the properties of GCD it is known that 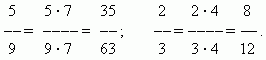



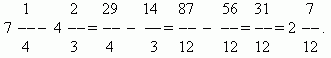









 Important! There is no need to rush to multiply the numerator and denominator of a fraction. After we have written down the product of the numerators of the fractions in the numerator, and the product of the denominators in the denominator, we need to factor each factor and reduce the fraction.
Important! There is no need to rush to multiply the numerator and denominator of a fraction. After we have written down the product of the numerators of the fractions in the numerator, and the product of the denominators in the denominator, we need to factor each factor and reduce the fraction.





 That is To divide by a fraction, you need to multiply by the "inverted" one.
That is To divide by a fraction, you need to multiply by the "inverted" one.